introduction
At a recent dinner, the dicussion turned to magnetic and electric fields as can happen when more than one electrical engineer is present – a confusing, yet enlighting phenomenan. One said something along the lines of “at fast speeds, the electric field just drops out of the B field”. Dazed, not because I had grasped the concept, but because nearly every word went past my head, I asked for clarification. “Due to Lorentz contraction….” the rest again a blur. Nodding, I knew the subject was too removed from any knowlege that I’d have to do some solitary leg work to get a glimpse of understanding.
B fields, E Fields, and Maxwell’s Equations
To break into this topic, I turned to ChatGPT and threw in some hot button words and asked for clarification. The prompt itself is a bit unintelligable, but the LLM was able to draft great first reply.
I’d like to learn about lorentz contraction and how the magnetic and electric fields are related and what they even are or represent. Can you please give me an overview of the concepts, starting first with the magnetic and electric fields. Give me equations (like Maxwells) that are fundamental for understanding these fields. ~ Message to GPT
Here’s a condensed version of what I gathered from the response – unfortunatly, and fortunatly, the human brain still needs to process the output from GPT to gain it’s own understanding.

Lorentz Contraction
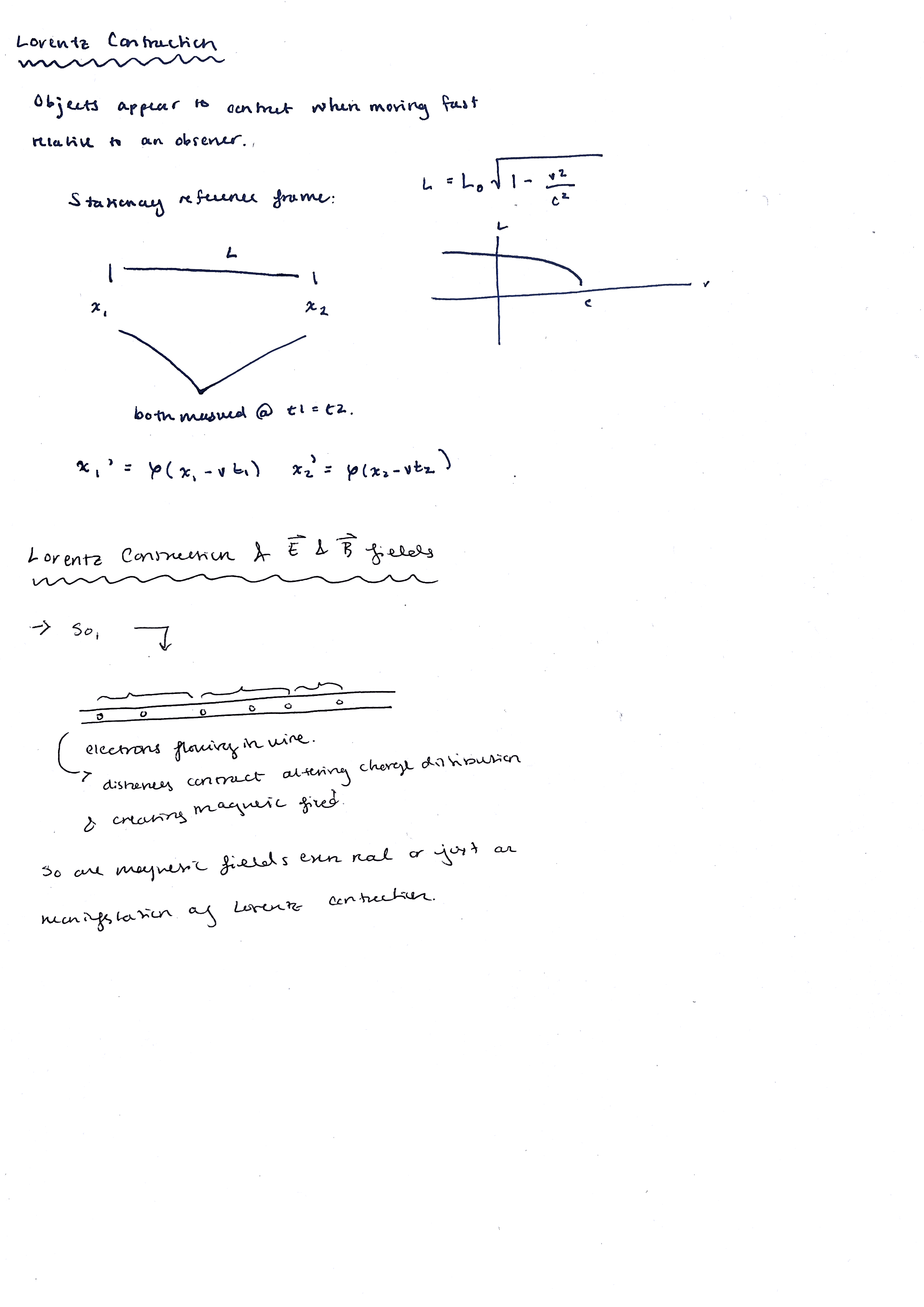
conclusion
My primary conclusion is that this field (who knows which one at this point) is wacky. These concepts will take a lot more time, thought, and repetition for me to sink in. However, this overview has given some guiding questions that maybe I will bring up if I am out at dinner with magnetical or electrical engineers in the future.
- Why does the distribution of point charges not effect the electric field surrounded by an arbitraty sphere encapsulating the charges. Is this an approximation of Maxwell’s equations?
- Is “induction” of a field equivalent to the curl of the electric and magnetic field. What is the magnitude versus curl of a field. What even is the magnitude of a vector field?
- If anybody would like, could we try to come up with examples of time dilation and contraction to build intuition for this? (Most likely this question may be met with people subtly saying “ah, let me get some more water” when their glass is already half full).
resources
- https://www.youtube.com/watch?v=-NN_m2yKAAk
- https://en.wikipedia.org/wiki/Gauss%27s_law
- https://en.wikipedia.org/wiki/Length_contraction
- https://en.wikipedia.org/wiki/Del
- plus other clickable links on Wikipedia around this topic